Patterns within systems of linear equations

This task will have you investigate linear equations in which the constants have a well-known

mathematical pattern.

PART A

Consider the following, linear equations whose constants follow a well-known pattern:

x + 2y = 4

25x + 5y = 1

3x + y = 1/3

2x – 6y = 18

• Examine the constants within each individual equation and describe the well-known pattern

common to the constants in each equation.

the constants in x + 2y = 4

are 1, 2, and 4

in the 2-nd, 25x + 5y = 1,

they are 25, 5, and 1,

in the 3-d, 3x + y = 1/3,

3, 1, and 1/3,

in the last equation, 2x – 6y = 18,

they are 2, – 6, 18.

One cannot help amazing at the well-known pattern common to the constants in each equation‼!

1 --> 2 --> 4

25 --> 5 --> 1

3 --> 1 -->1/3

2 --> – 6 --> 18

Isn’t it wonderful that geometric progressions with ratios 2, 1/5, 1/3, and – 3 appear in these equations in a mysterious way?!

Who stands behind this fact?

There is sure to be some intention because the constants exhibit the same well known pattern.

The pattern is the following:

x + qy = q²

How beautiful it is‼ What a wonderful world we live in‼!

• Give several other examples of equations fitting this pattern.

x + 3y = 9

36x + 6y = 1

4x + y = 1/4

x – 6y = 36

Rewrite these equations in the form y = mx + b

x + qy = q² --> y = – x / q + q,
i.e. m = – 1 / q and b = q

x + 2y = 4 --> q = 2, y = – x / 2 + 2

25x + 5y = 1 --> q = 1 / 5, y = – 5x  + 1 / 5

3x + y = 1/3 --> q = 1 / 3, y = – 3x  + 1 / 3

2x – 6y = 18 --> q = – 3, y = x / 3  – 3

• Write the general equations for this pattern of constants in both standard (Ax + By = C) form and slope-intercept (y = mx + b) form.

How are the constants A, B, C, m, and b related to each other? Explain why this is so.

B = Aq

C = Aq²

m = – 1 / q
b = q

In other terms,

B = Ab, C = Ab², mb = – 1

We have 2 degrees of freedom, say, A and q, or A and b, or A and m, or B and q, etc.

So each of the constants A, B, C, m, and b can be easily expressed in terms of these 2 degrees of freedom almost arbitrarily chosen.
The first degree of freedom must be among the constants A, B, C,
it explains the first term of the geometric progression,

but the 2-nd one among the constants m, q or b,
i.e. the value connected with the ratio of the geometric progression.

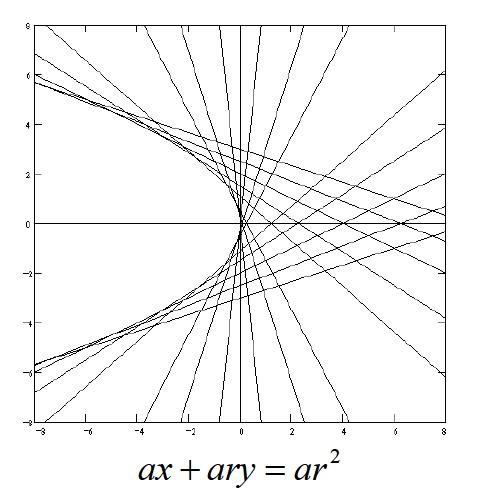
Now consider the entire family of linear equations fitting this pattern.

• On the same set of axes, graph a large sampling of representatives of this family, being sure to include a wide range of slopes. You may wish to explore this family further using the dynamic/ animated display features of your calculator or graphing software.

The slope m = – 1 / q

The intercept b = q

q being the ratio of the geometric progression

• Describe the graphical pattern you observe.

Nothing special is seen so far in this not very large sampling of representatives of this family,

so there is no point in graphing a larger sampling of this kind.

I do not think it is the place where “Arbeit macht frei”

Nothing special appears even after adding the coordinates of intersections.

• Now consider 2 x 2 systems consisting of pairs of equations fitting this pattern.

There are pairs of the equations
(the number of combinations where (unlike permutations) order does not matter),
i.e. there are 6 subsets of 2 distinct equations as elements of the set of 4 equations.

Table 1. 2 x 2 systems consisting of pairs of equations

Table 2. Solutions to the systems of pairs of equations

Table 3. Solutions to the systems of pairs of equations

It is still difficult to notice something interesting in these solutions.

• Create several systems of your own in which the equations exhibit the pattern observed

above. Solve your systems using at least two different methods, at least one of which should

involve the use of technology.

x + y = 1 (q = 1)

x + 2y = 4 (q = 2)

x + 3y = 9 (q = 3)

x + 4y = 16 (q = 4)

Solve our 1-st system

x + y = 1,

x + 2y = 4.

After subtraction we get y = 4 – 1 = 3.

So x = 1 – y = 1 – 3 = – 2.

We got solution (– 2, 3)

That is a great idea!

Table 4. Solutions to the systems of pairs of GOOD equations

As an example of using technology (rather silly in this case):

x + 2y = 4

x + 3y = 9

x =

y =

We got solution (– 6, 5)

• Describe the pattern you observe. Prove this relationship.

The pattern we observe is nothing but the old " well-known mathematical pattern", and namely geometric progressions.

If somebody thinks there is the relationship for the solution (– rs, r + s) to two linear equations whose coefficients follow a geometric progression with ratios r and s, they are strongly mistaken.

The relationship exists but it is hardly observable.

Let us suppose our IQ is high enough to observe the relationship for the solution (– rs, r + s) to two linear equations:

   x + ry = r²

   x + sy = s²

• Using your solution to the general system, or otherwise, provide a proof of the graphical pattern "observed" above.

After subtraction we get y = (r² – s²)/ (r – s) = r + s

So x = r² – ry = r² – r (r + s) = r² – r² – rs = – rs

Part B of Patterns within systems of linear equations

x + 2y = 3

25x + 5y = – 15

3x + y = – 1

2x + 6y = 10

- Examine the constants in the first equation (x + 2y = 3) and describe any patterns.

1 --> 2 --> 3

An arithmetic progression, is it not?

Repeat for the second equation 25x + 5y = – 15:
25 --> 5 --> – 15

How do I describe the patterns?

5 is halfway between 25 and – 15:

5 – 25 = – 15 – 5

An arithmetic progression again! Incredible‼

It is really a well-known mathematical pattern. What a success‼!

Consider the 2x2 system of linear equations:
x + 2y = 3
2x – y = – 4
To solve the system,
Multiply through the 1-st equation by 2 to get:
2x + 4y = 6
2x – y = – 4
------
Subtract the 2nd from the 1st and solve for "y":
5y = 10
y = 2
----
Substitute into 2x – y = – 4 and solve for "x":
2x – 2 = – 4
x – 1 = – 2
x = – 1

Consider the 2x2 system of linear equations:
2x – y = – 4
25x + 5y = – 15

x =

y =

We got solution (– 1, 2).

- Make a conjecture regarding this type of 2x2 system and prove it.
Hence we can conclude that for any 2x2 system of linear equations
whose coefficients follow an arithmetic progression,

the values of x and y will always be – 1 and 2 respectively.

It is a conjecture to be proved.

ax + (a + d)y = (a + 2d) => this is series 1: a, a + d, a + 2d

bx + (b + c)y = (b + 2c) => this is series 2: b, b + c, b + 2c

Multiply equation 1 by b, equation 2 by a, and subtract:

(db – ac)y = 2(db – ac)

If db – ac = 0, then a(b + c) = b(a + d)
and hence the equations are ill defined, i.e. linear-dependent.

If db – ac ≠ 0, then y = 2

Plug this back in to get x = – 1

- Using technology, extend your investigation to 3x3 systems whose constants exhibit the same patterns seen above
x + 2y + 3z = 4           (1)
x + 3y + 5z = 7           (2)
x + 5y + 9z = 13         (3)
subtract (2) – (1):

y + 2z = 3 => y = 3 – 2z
The same we get subtracting (3) – (1) or (3) – (2).

x = 4 – (2y + 3z) = 4 – (2(3 – 2z) + 3z) = z – 2

- Make a conjecture regarding the solution to those 3x3 systems and prove your conjecture..

Suppose the solution to those 3x3 systems if well defined is (z – 2, 3 – 2z, z).

Proof:

ax + (a + d)y + (a + 2d)z = a + 3d                 (1) => this is series 1: a, a + d, a + 2d, a + 3d

bx + (b + c)y + (b + 2c)z = b + 3c                 (2)

px + (p + q)y + (p + 2q)z = p + 3q                (3)

subtract a∙(2) – b∙(1):

(db – ac)y + 2(db – ac)z = 3(db – ac) => the two equations are ill defined or y + 2z = 3

And x + y + z = 1, if some two equations are well defined.

Moreover, the third equation will always be linear-dependent.

y = 3 – 2z

x = 1 – y – z = 1 – (3 – 2z) – z = z – 2.

So the solution to those 3x3 systems if well defined is sure to be (z – 2, 3 – 2z, z).